Birth-disaster process
X(t) is a continuous-time Markov process defined as follows:
- Each individual gives a birth after an exponential random
time of parameter λ, independent of each other.
- A disaster occurs randomly at exponential random time
of parameter δ.
- Once a disaster occurs, it wipes out all the entire population.
What is the infinitesimal generator matrix of the process?
Birth-Death processes
The slides for birth-death processes are available here.
On the octopus Paul (and 3)
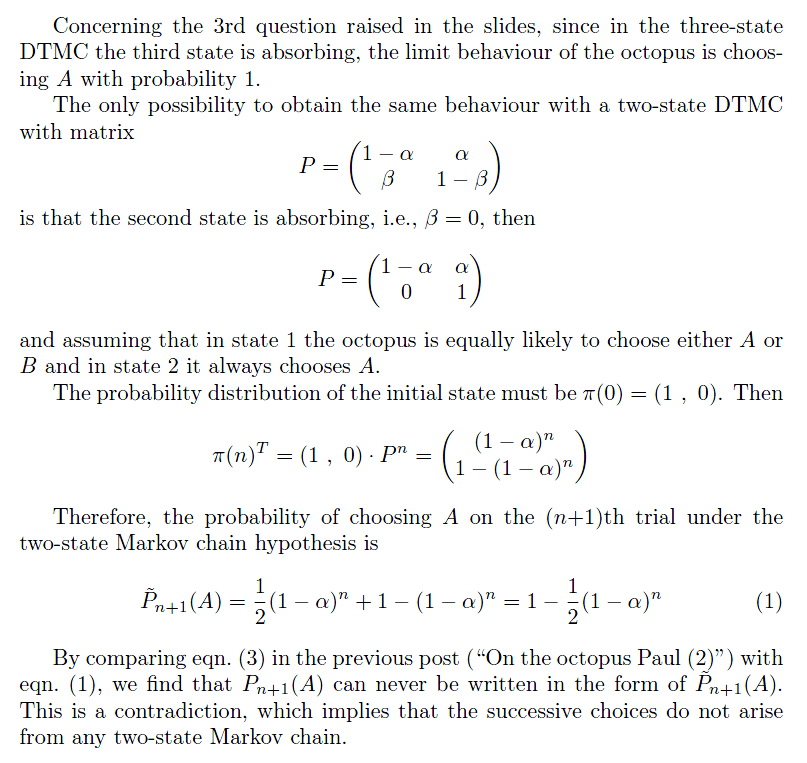
On the octopus Paul (2)

1 Technique not explained in the classroom, see for instance these notes.
On the octopus Paul (1)
 When I commented in the classroom the two first questions of the exercise of the octopus Paul (modelling its mind states with a DTMC), I misinterpreted the words “it is in state 1 just before…” as “it has been always in the state 1 before…”. Obviously, if we interpret the sentence correctly (“it is in state 1 at step n…”), we need to compute the transient probability distribution (after n steps), using the expression
When I commented in the classroom the two first questions of the exercise of the octopus Paul (modelling its mind states with a DTMC), I misinterpreted the words “it is in state 1 just before…” as “it has been always in the state 1 before…”. Obviously, if we interpret the sentence correctly (“it is in state 1 at step n…”), we need to compute the transient probability distribution (after n steps), using the expression
![]() I will publish here a solution for the exercise in the next days.
I will publish here a solution for the exercise in the next days.
CTMC slides updated
Today, we have updated the slides for the topic “Continuous Time Markov Chains. Applications in Bioinformatics”. You can find them here.
There is no class next Thursday (14th October)
Next Thursday (14th October) “is Monday” in the timetable of the Faculty of Engineering. Therefore, there is no class next Thursday 14th.
Preparando la web para el curso 2010-11
Estamos actualizando la web para el curso 2010-11…
Cambiando de aspecto
Estamos cambiando un poco el aspecto de estas páginas.